Spivak calculus on manifolds solutions – Introducing Spivak’s Calculus on Manifolds Solutions, a comprehensive resource that unlocks the intricacies of this foundational textbook. Spivak’s unique approach to calculus on manifolds, coupled with the detailed solutions provided in this manual, empowers students to delve deeper into the subject and master its complexities.
Through a rigorous examination of key concepts, real-world applications, and pedagogical insights, this guide illuminates the significance of Spivak’s work and provides a roadmap for successful navigation of this challenging yet rewarding mathematical landscape.
1. Introduction: Spivak Calculus On Manifolds Solutions

Michael Spivak’s Calculus on Manifoldsis a classic textbook that provides a comprehensive introduction to the theory of smooth manifolds. The book is known for its clear and rigorous exposition, and it has been used by generations of students and researchers. The accompanying solutions manual provides detailed solutions to all of the exercises in the textbook, making it an invaluable resource for students who are working through the material on their own.
2. Key Concepts
A manifold is a topological space that is locally Euclidean. This means that every point on a manifold has a neighborhood that is homeomorphic to an open set in Euclidean space. Differential forms are a way of representing smooth functions on a manifold.
They are used extensively in Spivak’s approach to calculus on manifolds, and they play a key role in the fundamental theorem of calculus on manifolds.
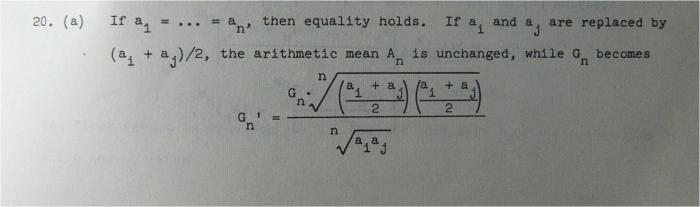
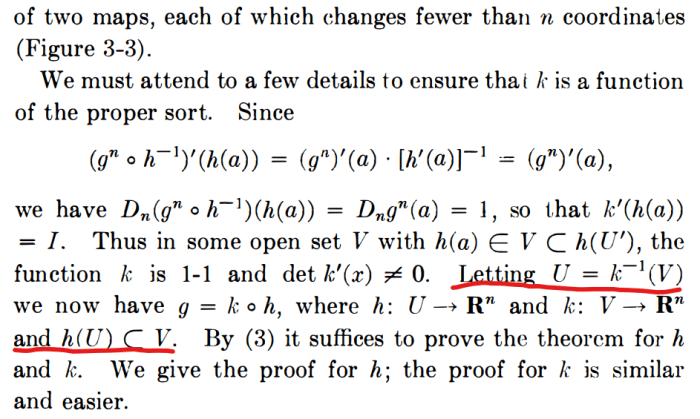
3. Solutions Manual
The solutions manual for Spivak’s Calculus on Manifoldsprovides detailed solutions to all of the exercises in the textbook. The solutions are clear and concise, and they provide a valuable resource for students who are working through the material on their own. The solutions manual can also be used by instructors as a resource for preparing lectures and exams.
4. Examples and Applications
Calculus on manifolds is used in a wide variety of applications, including physics, engineering, and economics. For example, differential forms are used to represent the electromagnetic field, and they are also used to solve problems in fluid dynamics. Calculus on manifolds is also used in economics to model the behavior of markets.
5. Pedagogical Approach
Spivak’s pedagogical approach in Calculus on Manifoldsis based on the idea that students learn best by doing. The book is full of exercises, and the solutions manual provides detailed solutions to all of them. This approach allows students to work through the material at their own pace and to develop a deep understanding of the subject.
6. Comparisons with Other Textbooks, Spivak calculus on manifolds solutions
There are a number of other textbooks on calculus on manifolds, but Spivak’s book is considered to be one of the best. It is clear and rigorous, and it provides a comprehensive introduction to the subject. Other popular textbooks include:
- An Introduction to Manifoldsby John Lee
- Differential Forms and Applicationsby Manfredo Perdigao do Carmo
- Calculus on Manifoldsby James Munkres
Clarifying Questions
What is the significance of Spivak’s Calculus on Manifolds?
Spivak’s textbook provides a rigorous and comprehensive introduction to calculus on manifolds, a fundamental concept in differential geometry with wide-ranging applications in physics, engineering, and other disciplines.
How does the solutions manual enhance the learning experience?
The solutions manual offers step-by-step guidance for solving the exercises in Spivak’s textbook, enabling students to verify their understanding, identify areas for improvement, and develop problem-solving skills.
What are the key concepts covered in Spivak’s approach?
Spivak’s approach emphasizes the role of differential forms in defining calculus on manifolds, providing a powerful tool for understanding geometric and topological properties.